Top 5 Tips For How To Solve Matrix Equations

Mathematics is not a difficult subject, but some topics are difficult to grasp. So, students are always scared of arithmetic and all its critical concepts. Matrix is one of them. Students often ask how to solve matrix equations and concepts.

But it is made easy if you understand the rationale and logic of mathematical concepts instead of getting up every maths question. 

Moreover, Matrix represents numbers or linear equations in block format to solve them. You can also add or subtract or multiply two or matrices with each other. This is why many students struggle with matrices and ask how to solve them. 

Here we will guide you through all the methods of solving the matrix easily with a proper step-by-step guide and best matrix equation examples.

What is Matrix?

Matrix is the main concept of mathematics. The meaning of a matrix is that it is a rectangular array of numbers, symbols, or expressions set in rows and columns. Matrices are generally used in various fields, such as mathematics, physics, engineering, computer science, etc.

A matrix is represented by having all its components within square brackets or parentheses. Moreover, the dimensions of a matrix are described by the number of rows and columns it contains. For instance, a matrix with two rows and three columns is called a 2×3 matrix.

Let’s know why the matrix is used. Matrices describe a vast range of mathematical objects and processes, such as systems of linear equations, geometry modifications, and many more. They also play an essential role in linear algebra, a branch of mathematics that deals with vector spaces and linear modifications.

Top 10 Types Of Matrix

You can calculate the matrix with the help of various types. These are the following types of Matrices.

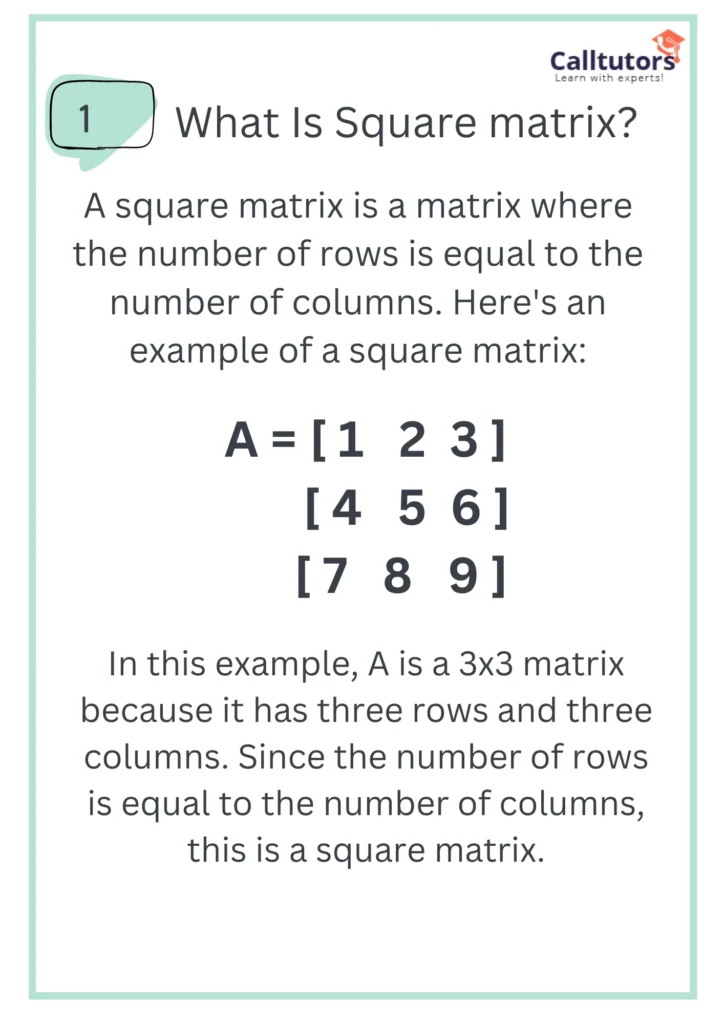
1. Square Matrix 

In mathematics, a square matrix is a matrix with the same number of rows and columns. An n-by-n matrix is known as a square matrix of order n. Any two square matrices of the same order can be added and multiplied. Square matrices often represent simple linear transformations, such as shearing or rotation.

2. Rectangular Matrix

A matrix is called a rectangular matrix if the number of rows is not equal to the number of columns.

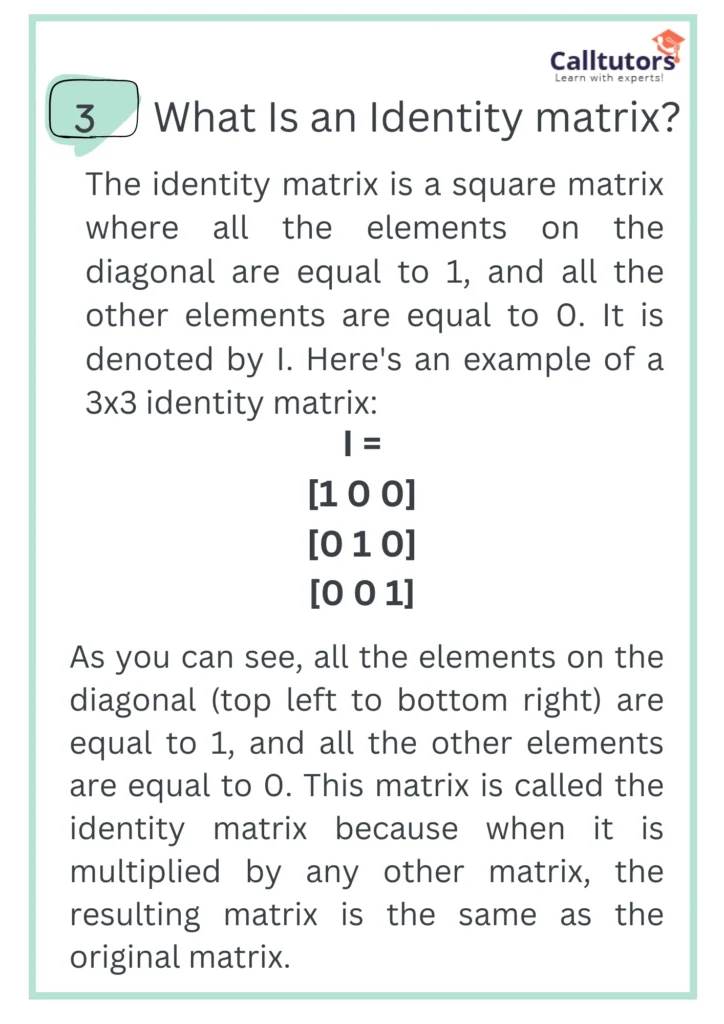
3. Identity Matrix

A square matrix in which all the diagonal elements are 1 and all other elements are 0 is called an identity matrix.

4. Zero Matrix

A matrix in which all elements are 0 is called a zero matrix.

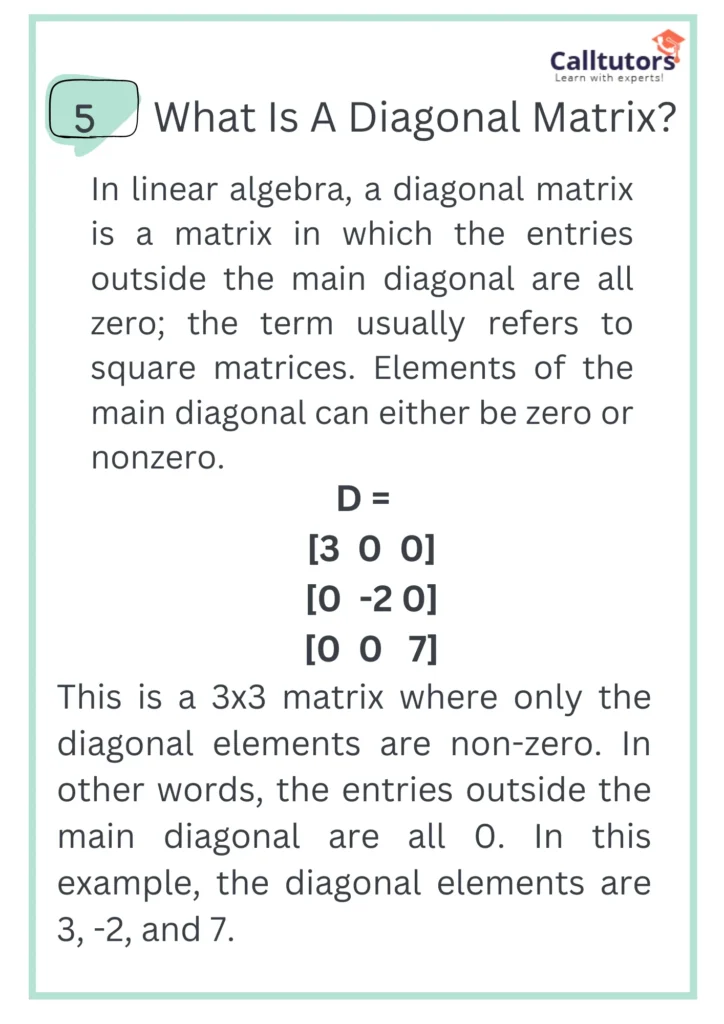
5. Diagonal Matrix

A square matrix in which all the non-diagonal elements are 0 is called a diagonal matrix.

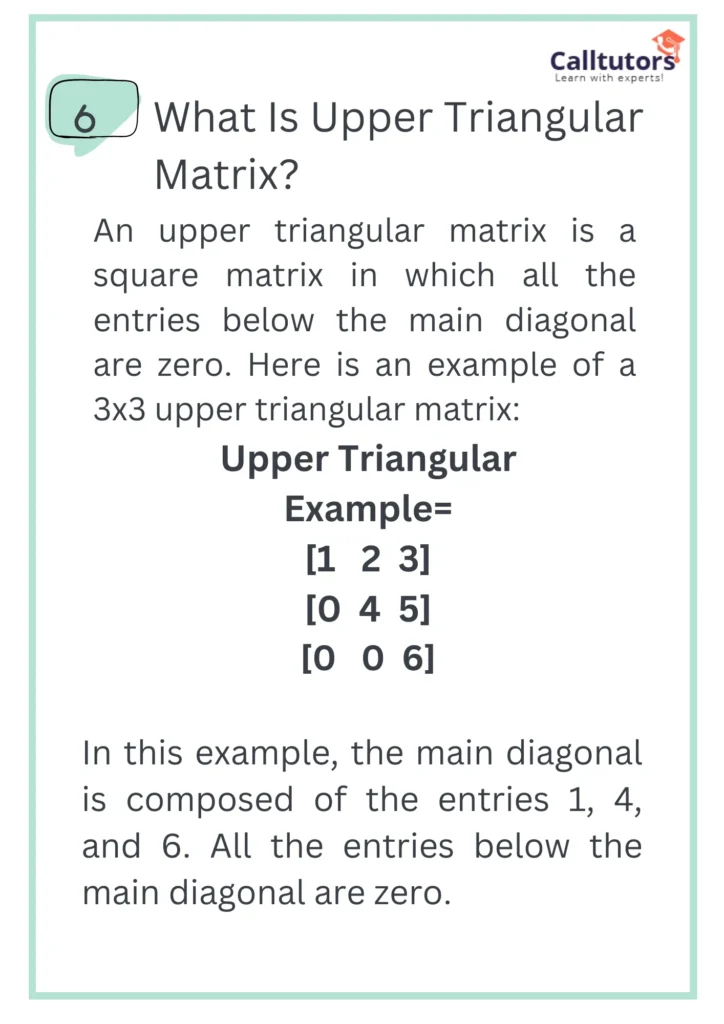
6. Upper Triangular Matrix

A square matrix in which all the elements below the main diagonal are 0 is called an upper triangular matrix.

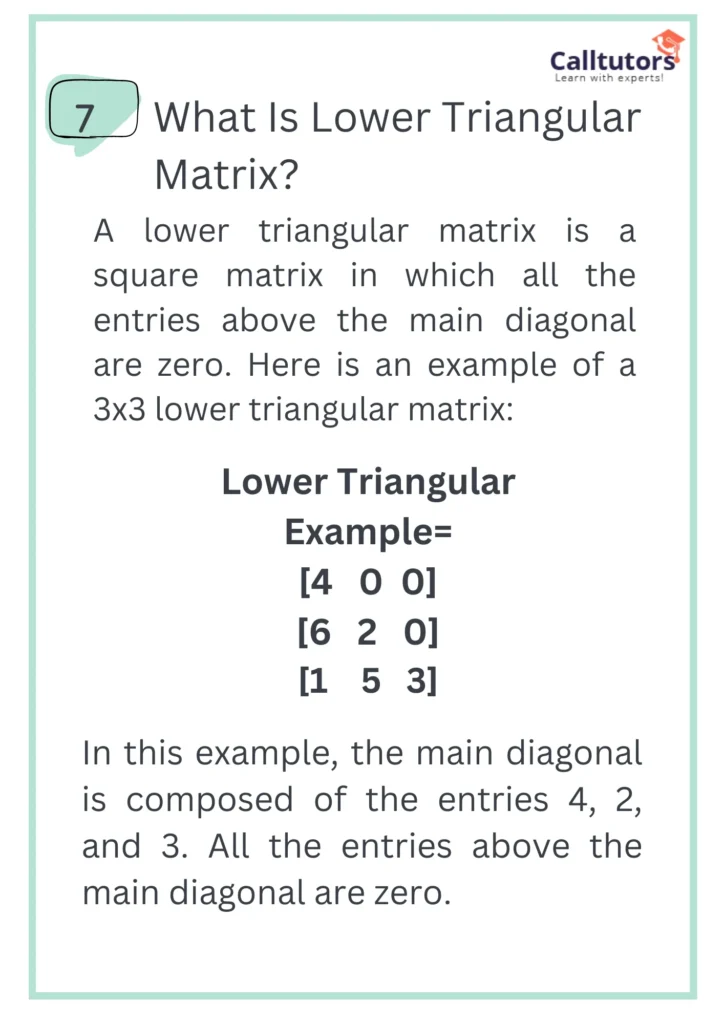
7. Lower Triangular Matrix

A square matrix in which all the elements above the main diagonal are 0 is called a lower triangular matrix.

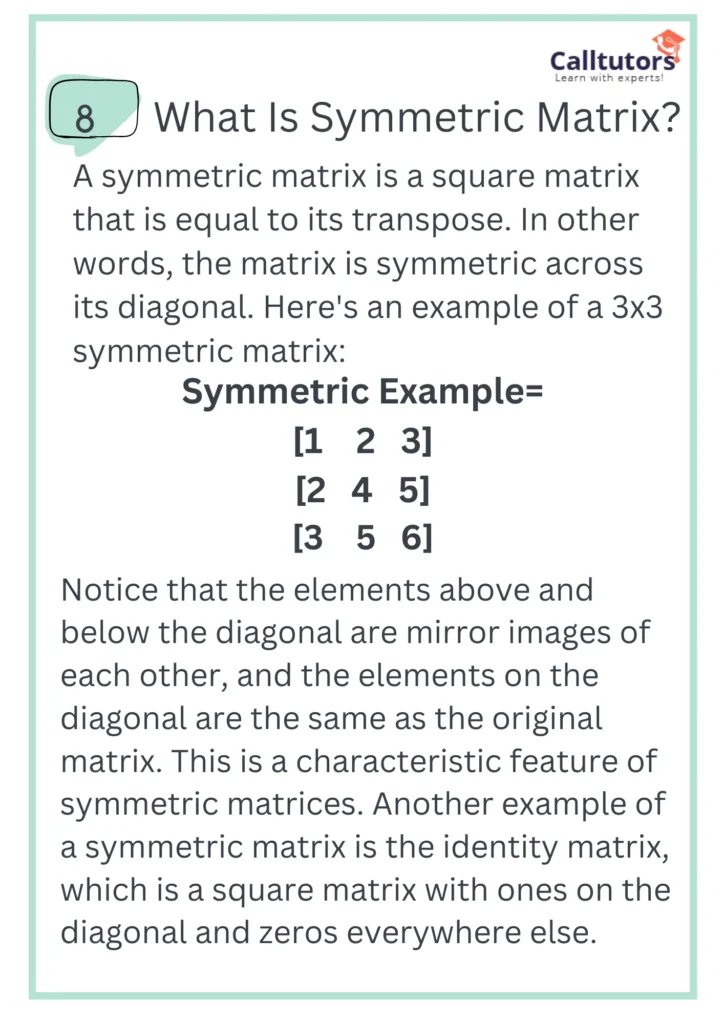
8. Symmetric Matrix

A square matrix in which the elements are symmetric with respect to the main diagonal is called a symmetric matrix.

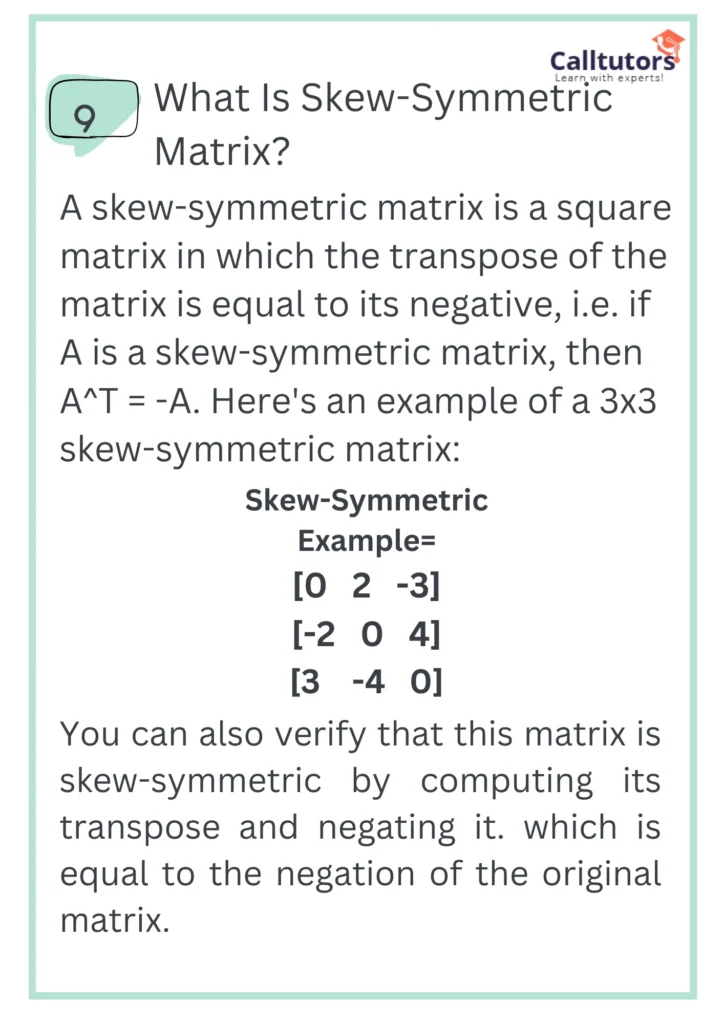
9. Skew-Symmetric Matrix

A square matrix in which the elements are skew-symmetric with respect to the main diagonal is called a skew-symmetric matrix.

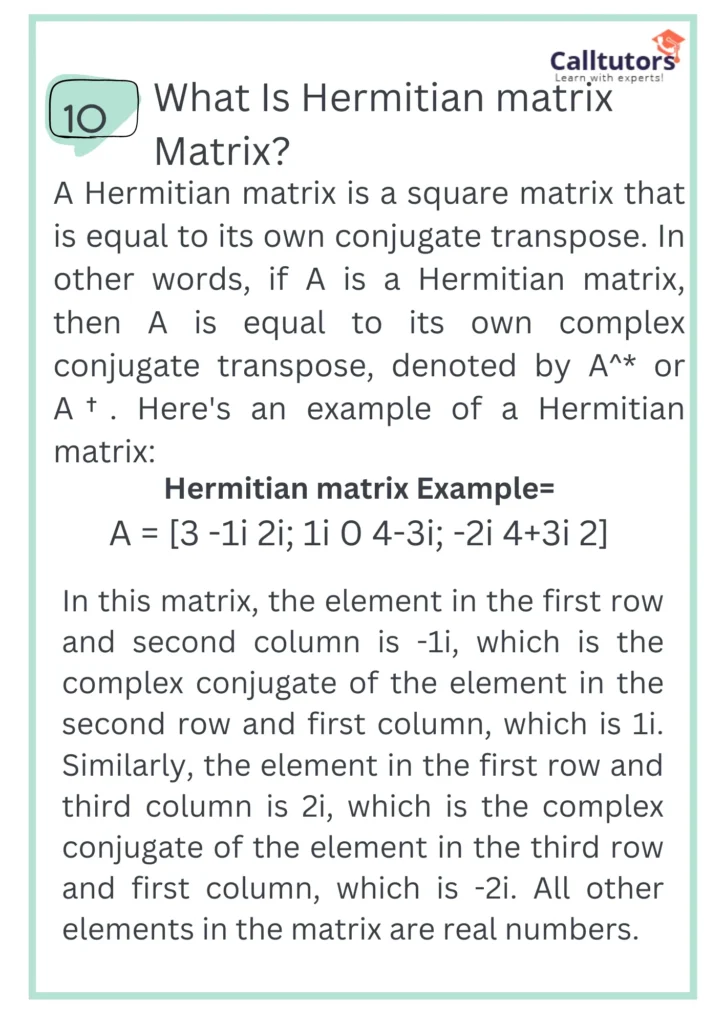
10. Hermitian Matrix

A complex square matrix in which the elements are symmetric with respect to the main diagonal and conjugate symmetric with respect to the conjugate diagonal is called a Hermitian matrix.

Top 5 Tips For How To Solve Matrix Equations With Examples

These are the following steps to solve the Matrix equations

1.  solving the matrix

The first step to solving the matrix is to check if you have sufficient data to find out each variable of the linear equation by using a matrix.

Matrix is used to solve the linear equation, but there should be more than one linear equation in order to use the matrix method. Thus if you have two equations only, then also you can use the matrix method to find out the variables.

Each linear equation has certain variables which we need to find out, and such variables have certain coefficients. Let’s understand it through given an example –

2x + 3y – 5z = 2

Here, x, y and z are variables of the equation that we need to find out, and +2, +3 and -5 are the coefficients in the equation.

2. write down your equations

Second step in How to solve the Matrix is to write down your equations in standard form calculator. Next question is what is the standard form of writing a linear equation then the answer is Ax + By + Cz = D.

So you have to write all the equations in such form in order to find out the values of variables using matrix.

It is not necessary that you have only 3 variables; there can be less than 3 variables and similarly there can be more than 3 variables. If you have more than 3 variables then you will add them in the left side after the z variable for example – Ax + By + Cz + Dw = E.

Here, A, B, C and D are coefficients and x, y, z, we are the variables.

3. transfer the data of the equation

The next step in How to solve Matrix is to transfer the data of the equation in matrix form. So the next thing to learn is how to write down equations in matrix form. Let’s us understand it through examples suppose we have following 3 equations –

x + 2y – 3z = 5

x + y + z = 6

2x + y – z = 1

Now we need to find out the variables using matrix format.  As we can see, the equations are already in standard form so we can proceed with transferring such equations into matrix form.

We write down the coefficients of each equation in row forms one after another. So here, we will first write down the  1, 2 and -3 in the first row of the matrix thereafter, we will write the coefficients of the second equation and then the 3rd equation. Look at the below to understand better –

Here, the rows and columns are also named. So we can name Row 1 as R1, which has 1, 2 and -3, Row 2 as R2, which has 1, 1 and 1 and similarly, row 3 as R3, which has 2, 1 and -1.

Likewise we can also name columns as C1, C2 and C3. C1 has 1, 1 and 2, C2 has 2, 1 and 1, and lastly C3 has -3, 1 and -1.

4. put large brackets around the first and last column

Next step in how to solve the matrix is to put large brackets around the first and last column to make it a matrix. Thus you will put square brackets [] around the whole block. It is just a symbol of matrices and nothing else you don’t have to worry or scare about the same.

5. Solve The Matrix

The next thing to learn in solving matrices is to add two matrices. You can add or subtract the two matrices.

Suppose the two matrices are

And second is

You can now add the matrices by adding R1 with the R1 of the second matrix and similarly all the numbers of the block.

Thus, you will get

Similarly, you will perform other functions like subtraction or multiplication etc.

Conclusion

We all get scared even with the name of mathematics. We struggle to solve most of the concepts and panic with the name of mathematics. Still, if we understand the logic of solving mathematical problems, we can easily solve all the problems irrespective of the complexity. Many students face problems in solving matrices. They also follow the steps and guides on how to solve matrices easily. Get the best help with math homework from the experts.

Frequently Asked Questions