In this blog, we are going to explain in detail about Kinematics Physics Equations, the important subject of Physics. This is a subject in which students find it very difficult to read because it uses many formulas and equations. Therefore, in this blog, we will tell kinematics physics equations very well to the students so that they can read it easily.
Mechanics have three branches namely kinematics, dynamics, and static. But in this blog, we will only elaborate on kinematics. Kinematics is the branch of mechanics where if something is moving, then you will want to know how it is moving.

Overview of a Kinematics
Table of Contents
Kinematics is the branch of mechanics in which you only want to know how the motion is moving and not want to know about why it is moving. In kinematics, students simply describe motion and do not want to know why and who is moving the motion.
In kinematics, we only want to know if an object is moving, where is the object moving, in which direction is it moving, what is the path of that object and how long is that object taking. It means to say that in kinematics we only describe the object and not to know why the object is moving.
As you all know that when describing motion in kinematics, there are four parameters for descending motion: displacement, velocity, acceleration, and time so that you can describe motion well.
Parameters Of Kinematics Physics Equations
Distance and Displacement
Distance and Displacement are the kinematics parameters by which we can understand motion. Displacement means a change in the position of any given object. Displacement tells us how much a given object is moving and how much it is moving in a given direction.

There is a difference between displacement and distance. In distance, we have to tell that the actual distance of the actual travel that we have done, and in the displacement, we have to tell that choosing the shortest route to get from one place to another place. Students are often confused about the meaning of these two, so we have explained the difference between distance and displacement.
Both distance and displacement are parameters of kinematics and help us to describe the motion of the given object.
The formula for displacement is as given below:
displacement=final position-initial position=change in position
S=Xf-Xi=change in X
Xf=final position
Xi=initial position
S=displacement
The formula for distance is as given below:
d=sum of the actual distance
Velocity
Kinematics has another parameter by which we can describe motion called Velocity. Velocity helps to describe the movement of motion. Velocity means how fast an object is moving from one point to another point in a given direction.
With the help of Velocity, we can find out the movement speed of an object. It is also the main parameter of kinematics physics equations. Velocity tells us how long an object is taking to move from one place to another so that we can estimate its speed.
Velocity Based On Speed and that gives us a proper average. With the help of velocity, we can know the speed of an object moving from one place to another and from which we find an average of it, which is called average velocity.
The formula for velocity is as given below:
v = Δs/Δt
Acceleration
Acceleration is a parameter of the kinematics physics equations, with its help we can also describe the speed. Acceleration means how much the velocity is changing every moment. This gives us an idea of how much accelerate a given object from one point to another point.
There is an inverse relationship between acceleration and time, if time increases then acceleration decreases, and if time decreases then acceleration increases.
The formula for acceleration is as given below:
a=Δv/Δt
Time
The importance of time is in everything, in the same way, there is a huge role of time in kinematics physics equations. Time is its parameter for describing motion, time is a single reference point for all the above parameters.
Time is used with almost every parameter. Without time we cannot describe the above three parameters. Therefore, there is a very important role of time in kinematic physics operations.
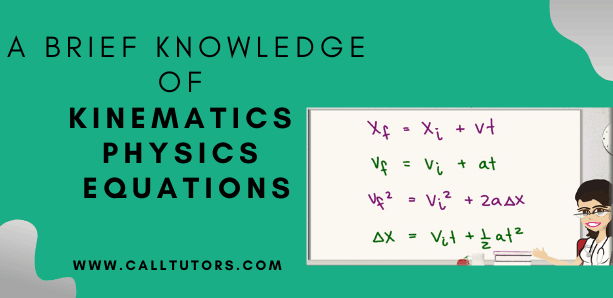
Kinematics Physics Equations
- v2=v1+aΔt
First of all, one must calculate the slope of the diagonal line. Here, the slope would be a change in velocity and divided by a change in time. Furthermore, the slope would equal acceleration.
a = v2–v1/t2–t1
One must rewrite t2 – t1 as Δt
a = v2−v1/Δt. This is certainly equation 1. One must rearrange it to get v2 on the left side. This would certainly express the formula in the slope-intercept form of a line.
v2 = v1 + aΔt
- Δx=(v+v0)t/2
To get the next formula, one must first derive an expression for the displacement of the object. Furthermore, the time interval is Δt. The calculation of the displacement is below:
S = vΔt
Furthermore, the displacement of the object is certainly equal to v1Δt. Also, the product v1 is equal to the area A1.
So A1 = v1Δt
Then, A2 = (V2−V1Δt)/2
Now adding A1 and A2
s = A1 + A2
Substituting for A1 and A2 gives
s = (v2−v1)/2Δt + v1Δt
Now simplifying it would give
s = (v2+v1)/2Δt. This is equation 2.
- s = v1Δt+aΔtsq/2
Equation no 3 is found out by eliminating v2
One must start with formula 1
v2 = v1 + aΔt
Now one must apply some algebra to make the left side of the formula to look like the right side of formula 2
v2 + v1 = v1 + aΔt+ v1
v2+ v1 = 2v1 + aΔt
Furthermore, one must multiply both the sides by 12Δt
s = (v2+v1)/2Δt= (2v1+aΔt)/2Δt
s = v1Δt+aΔtsq/2. This is formula 3
- v2sq= v1sq + 2as
Formula 4 is found by eliminating the time variable, or Δt
Now, one must certainly begin with equation 1 whose rearrangement has taken place with the acceleration on the left side of the equals sign
a = v2−v1/Δt
Furthermore, one must multiply the left side of equation 1 by the left side of equation 2. Moreover, one must multiply the right side of equation 1 with the right side of equation 2.
s = (v2+v1)/2Δt
as = [(v2–v1)/2Δt][v2−v1/Δt]
Then Δt cancels out which certainly leads to the simplification of the equation.
2as = v2sq−v1sq
This formula is almost always written as:
v2sq= v1sq + 2as. This is formula 4.
Quick Links

CONCLUSION
In this blog, we have explained the subject of physics in kinematics. Students are often confused about the kinematics physics equations because it uses a lot of formulas and equations, so we have defined the main formulas and equations of kinematics in this blog.
We hope you all came to know about Kinematics Physics equations. If there is still any doubt related to Kinematics Physics equations you will contact our experts, you will also get Physics Homework Help online or Physics assignment help from the experts in our calltutors you can get help.